The DEM-based treatment is extensively used in application to the optically thin EUV and X-ray emissions. There has been no matching treatment in the radio domain, where optical depth of emission can be big, and both free-free and gyroresonance thermal emission mechanisms are included.
Another important element that is often overlooked however can impact the free-free radio emission is the contribution of heavy (highly-ionized) ions. Abundances of heavy aspects relative to Hydrogen are reasonably small, their contribution to the free-free emission can be substantial due to dependence of the emission on Z2 (the charge square). This contribution depends on both the plasma composition (i.e., the component abundances) and the temperature (i.e., the ionization states).
Method
We have actually extended the “classical” theory of the thermal free-free and gyroresonance radio emission to the case of a multi-temperature plasma; the brand-new theory has actually been carried out in a freely-available computer code (Kuznetsov et al. 2021). For the free-free emission, the new theory and code include:
There has been no matching treatment in the radio domain, where optical depth of emission can be large, and both free-free and gyroresonance thermal emission systems are involved. Abundances of heavy elements relative to Hydrogen are relatively small, their contribution to the free-free emission can be considerable due to reliance of the emission on Z2 (the charge square). In Figure 3, we compare the free-free and gyroresonance radio emission spectra computed utilizing the new multi-thermal technique and the corresponding (DDM-based) isothermal approximation. For both emission mechanisms, the multi-thermal plasma produces substantially higher emission fluxes, due to the contribution of particles with above-average energies; the distinction is up to an element of ~ 1.5 for the free-free emission and up to an element of 2-6 for the gyroresonance emission. For the free-free emission, we have actually likewise considered the component abundances typical of either the solar corona or the photosphere/chromosphere; in the corona, due to higher abundances of low-FIP heavy components (mostly– iron) and for this reason stronger contribution of highly-ionized ions, the emission strength is greater by up to 10%.
.
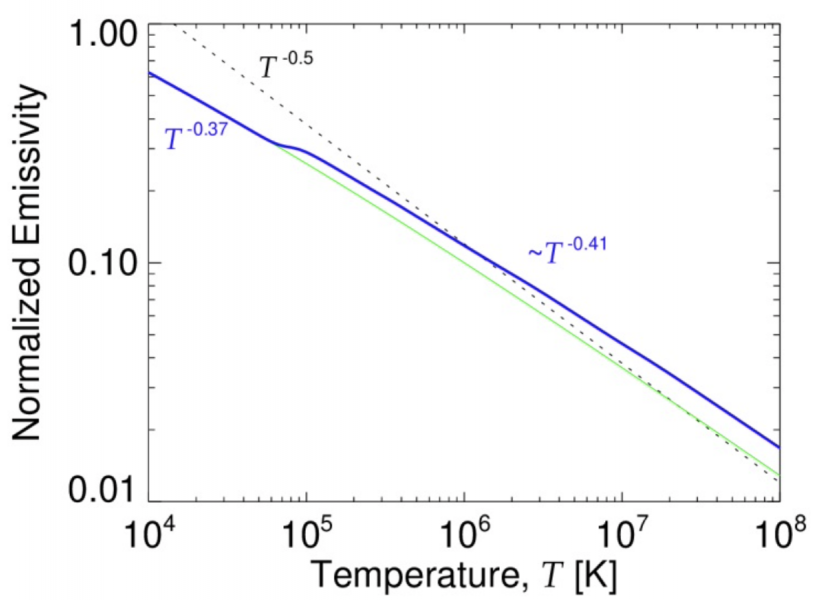
Figure 1– Dependence of the free-free emissivity at 1 GHz on temperature: green line shows the outcome for a hydrogen plasma taking into consideration the specific Gaunt aspect, while the blue line also represents the temperature-dependent ionization of heavier elements in the solar corona. For contrast, the dotted line shows the T-0.5 dependence.
For the gyroresonance emission mechanism, we have actually introduced a new procedure– the differential density metrics (DDM), which represents a circulation of the thermal electron density over temperature level. The new theory and code utilize this DDM to compute the gyroresonance radio emission from a multi-temperature plasma (the gyroresonance system is insensitive to the component abundances or Gaunt element).
To our previous numerical tools for simulating the solar radio emission (Fleishman & & Kuznetsov 2010; Fleishman & & Kuznetsov 2014), the new computer code computes the radio emission from one or more lines-of-sight by resolving the radiation transfer formula. When the emission crosses quasi-transverse magnetic field regions, the code likewise accounts for possible depolarization or polarization turnarounds.
The new theory is provided in a paper recently published in ApJ (Fleishman et al. 2021). The corresponding computer system code is implemented as executable libraries callable from IDL; it is readily available on GitHub and in the Zenodo repository (Kuznetsov et al. 2021).
Results.
Figure 2 shows an example of DDM and DEM circulations acquired from an upgraded EBTEL code (thanks to J. Klimchuk; personal communication); these distributions will be used below to calculate the model emission spectra. The corresponding average plasma temperatures (computed as minutes of the distributions) are of about 2.6-2.8 MK, however there are substantial quantities of particles with the temperature levels significantly above the average worths.
Figure 2– Model DDM and DEM distributions (courtesy of Jim Klimchuk) utilized in the simulations. The distributions represent the plasma heating specifications typical of a solar active area
.
Figure 3– Model radio emission spectra computed using the electron circulations displayed in Figure 2. a) Free-free emission (no electromagnetic field); b) gyroresonance emission (magnetic field varying linearly with height from 1000 to 300 G along the line of sight). The emissions were computed both for the multi-thermal DEM/DDM approach and for an isothermal case corresponding to DDM-averaged temperature and density.
In Figure 3, we compare the free-free and gyroresonance radio emission spectra calculated using the brand-new multi-thermal approach and the matching (DDM-based) isothermal approximation. For both emission systems, the multi-thermal plasma produces substantially higher emission fluxes, due to the contribution of particles with above-average energies; the difference depends on an element of ~ 1.5 for the free-free emission and up to an aspect of 2-6 for the gyroresonance emission. For the free-free emission, we have actually likewise thought about the component abundances typical of either the solar corona or the photosphere/chromosphere; in the corona, due to greater abundances of low-FIP heavy elements (mostly– iron) and hence stronger contribution of highly-ionized ions, the emission strength is higher by approximately 10%.
Extra information: Based on the recent paper by Fleishman, Kuznetsov, & & Landi, “Gyroresonance and Free-Free Radio Emissions from Multithermal Multicomponent Plasma”, ApJ, 914, 52 (2021 ). DOI: 10.3847/ 1538-4357/ abf92c.
References.
Fleishman, G.D., Kuznetsov, A.A.: 2010, ApJ, 721, 1127.
Fleishman, G.D., Kuznetsov, A.A.: 2014, ApJ, 781, 77.
Fleishman, G.D., Kuznetsov, A.A., Landi, E.: 2021, ApJ, 914, 52.
Kuznetsov, A., Fleishman, G., Landi, E.: 2021, Zenodo:4625572.
* Full list of authors: Alexey Kuznetsov, Gregory Fleishman, and Enrico Landi.
the multi-temperature plasma structure (described by the DEM), which is used to both optically thin and thick emissions;
the temperature-dependent ionization of the elements making up the plasma (which consists of the reliance on the element abundances), see Figure 1;
exact worths of the Gaunt element which figure out the Coulomb logarithm, see Figure 1;
contributions of electron accidents with both ions and neutral (hydrogen and helium) atoms;
effect of the ambient magnetic field